This webpage discusses an article written in 2004 by McKinsey on the IRR and demonstrates that the critiques are wrong. McKinsey makes a critique of using the IRR in terms of capital investment decisions. They make the point that the IRR can result in a ranking of investment decisions that is different from the ranking from a fundamental NPV rule. McKinsey makes the point that as the IRR implicitly assumes that intermediate cash flows are re-invested at the IRR itself, it is possible come up with situations where the IRR criteria and the NPV criteria produce different results. You can find these points made by McKinsey in the link below:
The McKinsey article authors make a dramatic accusation: “For decades, finance textbooks and academics have warned that typical IRR calculations build in reinvestment assumptions that make bad projects look better and good ones look great. Yet as recently as 1999, academic research found that three-quarters of CFOs always or almost always use IRR when evaluating capital projects.” McKinsey continues: “Our own research underlined this proclivity to risky behavior. In an informal survey of 30 executives at corporations, hedge funds, and venture capital firms, we found only 6 who were fully aware of IRR’s most critical deficiencies. Our next surprise came when we reanalyzed some two dozen actual investments that one company made on the basis of attractive internal rates of return. If the IRR calculated to justify these investment decisions had been corrected for the measure’s natural flaws, management’s prioritization of its projects, as well as its view of their overall attractiveness, would have changed considerably.”
McKinsey advocates use of NPV rather than IRR and use of the MIRR to fix the famous re-investment problem. In the discussion below I explain the article is largely rubbish. I explain that the MIRR does not fix anything at all and the WROI would be a much better ranking method. I begin with a five year cash flow case used in the McKinsey article.
Ranking of Investments with the Same Life
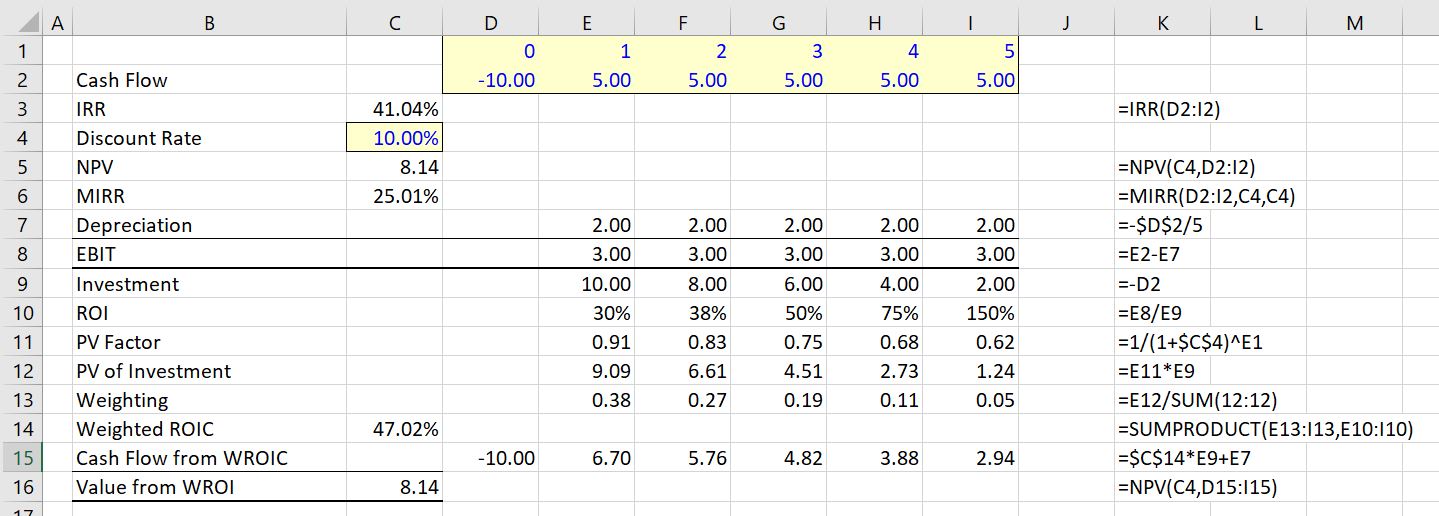
To illustrate how the IRR can distort ranking of investments begin with the example used in the McKinsey article. This case has an outlflow of 10 followed by positive 5 cash flow for 5 years. This cash flow produces an IRR of 41.04%. With a discount rate of 10%, the MIRR is 25.01% and the weighted average ROI is 47.02%. The reconciliation of the NPV of 8.14 is confirmed with the NPV from the weighted ROI in the screenshot below. When you would just add up the 5 years of 5 cash flow, you would get 25. McKinsey correctly complains that the IRR on this investment with that produces 25 cash flow is higher than another investment when you have to wait five years to receive 50 in cash flow.
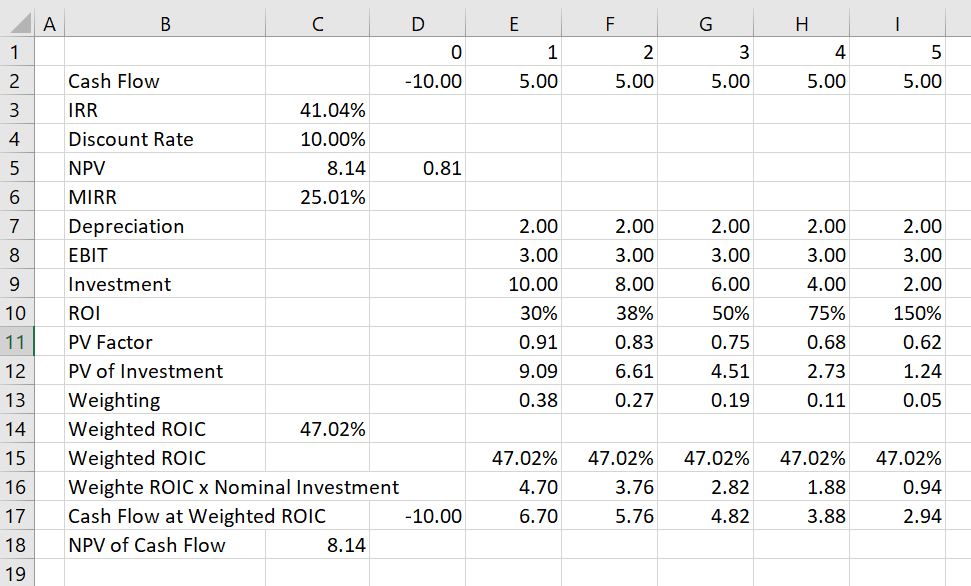
Next consider a case where there are no middle year cash flows and the final cash flow in period is 50. The cash flow of 50 is much more than the 5 years of cash flow of 5 that adds up to 25. But because of timing, the un-adjusted IRR is lower than the first case. The IRR is 37.97% as shown in the screenshot below. But when the NPV is computed at a discount rate of 10% the NPV of the investment is higher — it is 19.13 rather than 8.14. This is thus a classic case of different ranking for NPV and IRR. The first investment with 5 years and a cash flow of 5 has a better ranking from the IRR than the investment shown in the screenshot below. But the first investment above has a lower ranking than the investment shown below when the NPV is used. The correct decision would be to use NPV and take the second investment.
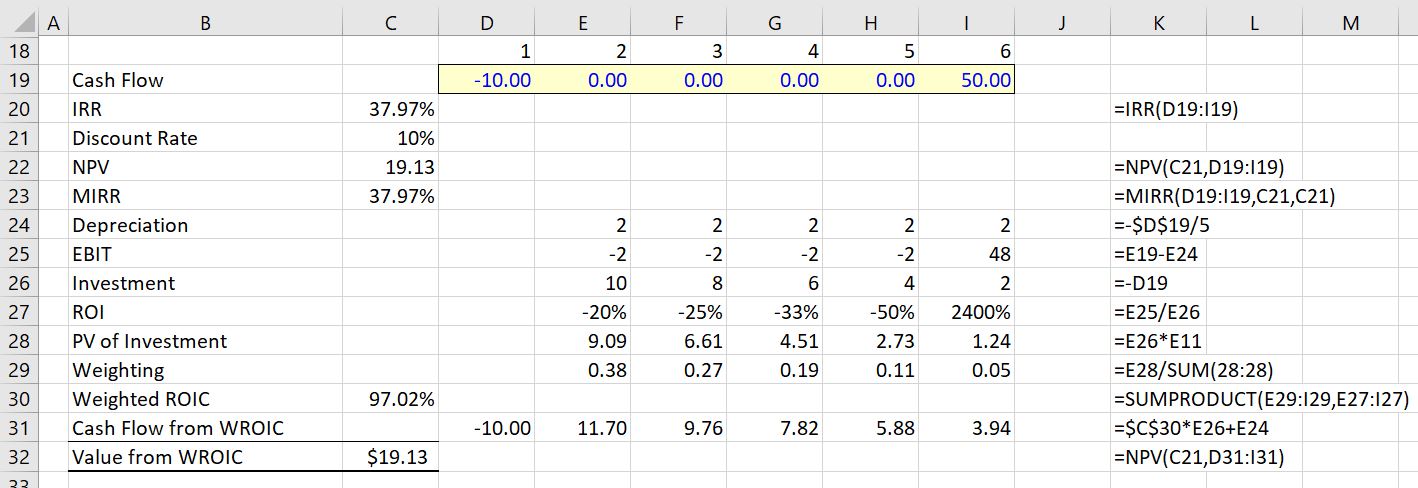
When looking at the above screenshot, you can see that MIRR of 25.01% is lower for investment 1 than for investment 2 where the MIRR is the same as the overall IRR of 37.97%. The MIRR is the same in the second case because there are no middle period cash flows. In the first case, the MIRR is lower than the IRR because the middle cash flow earns a lower return than the overall IRR. In terms of the WROI, the ranking is the same as the correct ranking where the second investment has a very high WROI. The MIRR of 25.01% for the first investment is lower than any of the ROI.
When the calculated IRR is higher than the true reinvestment rate for interim cash flows, the measure will overestimate—sometimes very significantly—the annual equivalent return from the project. The formula assumes that the company has additional projects, with equally attractive prospects, in which to invest the interim cash flows. In this case, the calculation implicitly takes credit for these additional projects. Calculations of net present value (NPV), by contrast, generally assume only that a company can earn its cost of capital on interim cash flows, leaving any future incremental project value with those future projects.
The McKinsey article discusses the problem with the following jiberish: “IRR’s assumptions about reinvestment can lead to major capital budget distortions. Consider a hypothetical assessment of two different, mutually exclusive projects, A and B, with identical cash flows, risk levels, and durations—as well as identical IRR values of 41 percent. Using IRR as the decision yardstick, an executive would feel confidence in being indifferent toward choosing between the two projects. However, it would be a mistake to select either project without examining the relevant reinvestment rate for interim cash flows. Suppose that Project B’s interim cash flows could be redeployed only at a typical 8 percent cost of capital, while Project A’s cash flows could be invested in an attractive follow-on project expected to generate a 41 percent annual return. In that case, Project A is unambiguously preferable.”
“Even if the interim cash flows really could be reinvested at the IRR, very few practitioners would argue that the value of future investments should be commingled with the value of the project being evaluated. Most practitioners would agree that a company’s cost of capital—by definition, the return available elsewhere to its shareholders on a similarly risky investment—is a clearer and more logical rate to assume for re-investments of interim project cash flows (Exhibit 1).”
Case with Projects that have Different Lives Demonstrating Problems with MIRR advocated by McKinsey
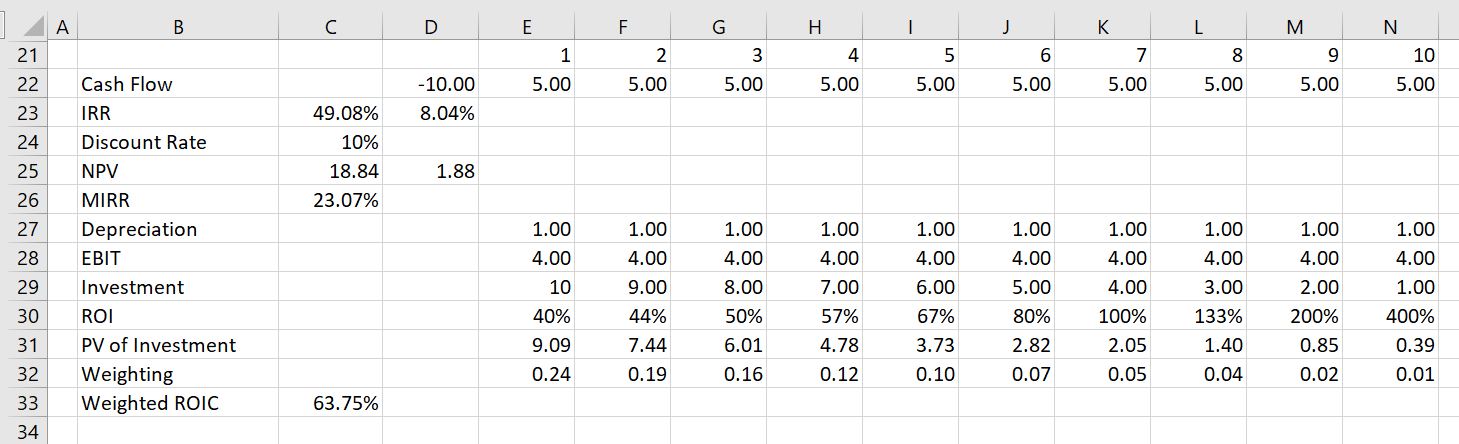
When investments with different lives are ranked, the MIRR falls apart as a statistic. On the other hand, the weighted average ROI continues to correctly value different projects. To illustrate this, compare the case with 5 years of 5 cash flow to a case with 10 years of 5 cash flow. If the investment is the same, it is pretty obvious that you would rather have the longer cash flow. But the MIRR gives a lower return to the longer cash flow because it compresses cash flows to the re-investment rate. The longer term cash flow project would be ranked lower than the project with shorter cash flow.
To illustrate this case, return first to the 5-year case. This is a repeat of the screenshot above with an IRR of 41.04% with an MIRR of 25.01% and a weighted ROIC of 47.02%. The NPV of the investment is 8.14.
In the second case where the cash flows are simply extended, the IRR increases to 49.08% (not too much) and the MIRR declines from 25.01% to 23.07%. The NPV of the investment more than doubles from 8.14 to 18.84. Thus, the MIRR would actually suggest that the investment below is worse than the investment with only 5 years of cash flow. The MIRR is not consistent with the NPV. The NPV is B.S. On the other hand, the WROI increases dramatically as did the NPV. It increases from 47.04% to 63.75%.
Videos that Describe WROI as an Alternative to MIRR or IRR
The three videos below describe the problems with IRR when you have middle period cash flow. It is demonstrated that a weighted average return solves problems with the IRR in terms of measuring growth and ranking investments.
Comments on IRR
I purchased your book several years ago and just stumbled on your website/youtube videos the other day. Your latest videos on IRR/MIRR caught my attention as I’ve had the same arguments regarding IRR several times, and your WROIC is interesting. I messed around with the excel document and it seems to improve on some shortcomings of IRR and NPV. It does a better job capturing investment life (with equal NPVs, equal investment, a shorter investment life gives a higher WROIC), and investment amount (with equal NPVs, equal life, a lower investment yields a higher WROIC), and having that captured in a single metric is very useful for a management team that wouldn’t usually be familiar with the details of various projects/investments under consideration.
Getting management teams on board always seems to be the greatest challenge, and one thing I see causing confusion is the Depreciation, as in this calculation it doesn’t necessarily tie to either Tax or Book depreciation, but just the project/investment life. I haven’t tried the WROIC calculation in a full model with multiple investment periods, but while the NPV of Cashflow at Weighted ROIC is fine when you just add another period of negative cash flow, the Weighted ROIC is incorrect. Starting the calculation following the last investment period seems to solve the problem, is that how you recommend doing it?
That McKinsey article is utter garbage, but unfortunately has been cited numerous times. IRR certainly has problems, but whoever wrote the advice the article provides is beyond terrible. Like I mentioned I’ve had the “IRR is only accurate when proceeds can be reinvested at the same rate” argument several times and the best way I’ve seen to that isn’t correct is the example below. Create a simple project model and solve for the IRR, then create a bank account earning interest at the IRR of the project, where negative cashflows are deposits and positive cashflows are withdrawals. It’s clear here that your account balance is earning the IRR, and the cashflows taken out doesn’t need to be reinvested anywhere.
| Project/Investment | |||
| Period | 0 | 1 | 2 |
| Investment | (100) | ||
| Return | – | 130 | |
| Total Cashflow | (100) | – | 130 |
| IRR | 14.02% | ||
| Bank Account Earning Interest at IRR | |||
| Account Balance | 100.00 | 100.00 | 114.02 |
| Interest Earned | – | 14.02 | 15.98 |
| Account Balance End of Period | 100.00 | 114.02 | 130.00 |
| Withdrawal | – | – | (130) |
| Account Balance After Withdrawal | 100.00 | 114.02 | (0.00) |
| Project/Investment | |||
| Period | 0 | 1 | 2 |
| Investment | (100) | ||
| Return | 160 | 70 | |
| Total Cashflow | (100) | 160 | 70 |
| IRR | 95.76% | ||
| Bank Account Earning Interest at IRR | |||
| Account Balance | 100.00 | 100.00 | 35.76 |
| Interest Earned | – | 95.76 | 34.24 |
| Account Balance End of Period | 100.00 | 195.76 | 70.00 |
| Withdrawal | – | (160) | (70) |
| Account Balance After Withdrawal | 100.00 | 35.76 | (0.00) |
The other thing I’ve shown people is how to replicate the MIRR function by just trapping all the positive cashflow from a project, earning the reinvestment rate on the cumulative balance, and releasing it at the end of project life. That makes it pretty clear that MIRR isn’t really a Modified IRR, but it really modifies the project cashflows and then does the same calculation as a regular IRR.